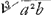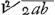Oeuvres complètes. Tome III. Correspondance 1660-1661
(1890)–Christiaan Huygens–
[pagina 342]
| |
No 896.
| |
The Refutation.The Authors first mistake is in thinking that FG doth passe through H. And the ground of that mistake is an other that immediatly followes viz. That BN, is to BK; as NF to FK: and consequently that HN, is to HK; as NF, to FK. and therefore that HF diuides the Angle NHFGa naar voetnoot2) into two equal parts, and continued diuides also the Angle LHO in the same manner; and therefore must cut AB at G, so that GB must be equal to BF. But NF, is to FK (not as BN to BK; or as HN to HK; but) as BN is to BF; or BF, to FKGa naar voetnoot3). For the immediat differences of proportionals, are as the immediat proportionals themselues. Ergo &c. | |
And by way of instance.Let BE = 1. BA = 64. then must BF = 8. for 1. 8. 64. ∺ and therefore BH = √32 = 4 √ 2. But BK = 4. and BL = 16. for 1. 4. 16. 64. ∺ And LB + BK. LB (∷ LK. LH) ∷ KB. HR ∷ 5. 4 ∷ 4. 16/5. therefore HR = 16/5. wherefore HB = 16/5 √ 2. Therefore 4 √2 = 16/5 √2. and 4 = 16/5. or 20 = 16. which are impossible. Ergo &c. | |
Or, uniuersally.Let BE = a. BA = b. then must BF = √ab. for a. √ab. b. ∺ and therefore BH = ½ √2 ab. But BK = √c, a2b.Ga naar voetnoot4) and BL = √c, ab2 for a. √c, a2b. √c, ab2. b. ∺ And LB + BK. LB (∷ LK. LH)Ga naar voetnoot5) ∷ KB. HR ∷ √c, b + √c, a. √c, b ∷ ∷ √c, a2b. √c, a2b2/√c, b + √c, a | |
[pagina 343]
| |
therefore HR = √c, a2b2/√c, b + √c, a. wherefore √c, a2b2/ √c, b + √c, a √ 2 = HB. Therefore ½ √q, 2ab=√c, a2b2/√c, b + √c, a√2.Ga naar voetnoot6) therefore √ q, ab=2√c, a2b2/√c, b + √c, a therefore ab= 4ab √c, ab/√c, b2 + 2 √c, ab + √c, a2. therefore √c, b2 + 2 √c, ab + √c, a2 = 4 √c, ab. therefore √c, b2 - 2 √c, ab + √c, a2 = 0. therefore √c, b - √c, b - √c, a = 0. therefore a = b. Therefore in all other cases it is impossible. Ergo &c. |
|