Oeuvres complètes. Tome III. Correspondance 1660-1661

N^o 895.
[Th. Hobbes] au Roi Charles II.
Appendice II au No. 893.

La copie se trouve à Leiden, coll. Huygens¹⁾.

1) Cette copie est de la main de W. Brouncker.

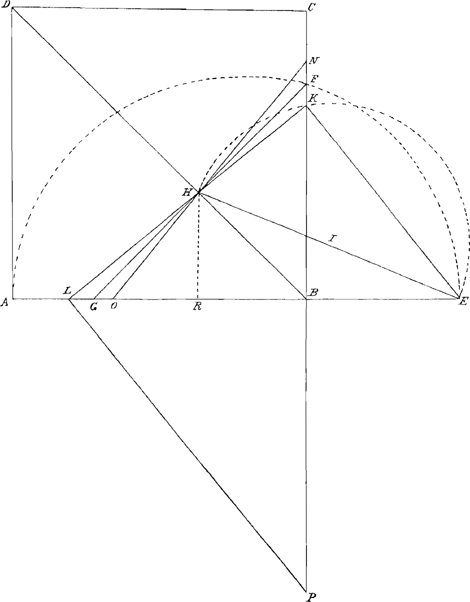
To find two mean Proportionals between two streight lines giuen.

Let AB be the greatest extream the square where-off is ABCD. Produce the side CB to P, so that CB, BP be equal. From P draw PL at adventure, cutting the side BA in L. Then draw perpendicularly to PL, the line LK, cutting the side BC in K. Again from K draw KE, perpendicular to LK, cutting AB produc'd in E. And so you haue, by construction, four continual proportionals, whereof the two meanes are BL and BK; and BP (equal to BA,) and BE the two extreames.

Between AB the greatest, and BE the least extream, find the mean-proportional BF, and BG equal to it; plac'd on the two sides BC and BA. and draw the diagonal DB, cutting LK in H; and diuiding the angle KBL into two equal parts. And so LH will be to HK, as LB the greater to BK the lesser of the meanes; also so will be AB to BL, and BK to BE.

On the center H, at the distance HL, describe an Arch of a Circle, cutting BC

[p. 340]

[p. 341]

in N; and BN, BL will be equal. And as HN to HK, so will be BN to BK; because HN & HL are equal.

Now BF (being a mean Proportional between AB & BE the Extreames) is also a mean Proportional between BN & BK the meanes. Therefore taking in BA the line BO, equal to BK, and joyning NO; the line NHO will be a streight line, and the Angles NHK, LHO vertical²⁾, and the line FG will passe through H.

And as BN to BK, so is NF to FK³⁾ (for the differences of proportionals are as the proportionals themselues) and so also HN to HK. and therefore the line FG diuides both the Angles NHK, LHO into equal parts.

Now BF the mean between the Extreames is known, therefore also FG is known, & the halfe of it BH is known both in magnitude & position, as being in the diagonal. Therefore the point H is known. lastly the point E is known by construction.

Draw EH and diuide it equally in I; and at the distance IH, or IE describe a semicircle. this semicircle will passe through K, because the Angle HKE is a right angle. Therefore the line BK, which is the lesser of the meanes, (and by consequence also the greater) is known.

2) Lisez: equal.

3) C'est ici que Hobbes se trompe.

The Construction of the Probleme.

Let the two extreams giuen be AB the greater, and BE the lesser. between which J am to find two meanes in continual proportion. Vpon AB the greater extream, J describe a square ABCD, and draw the diagonal BD; in which take BH equal to halfe that streight line whose square is double to the square of a mean proportional between the extreames giuen. then joyning the points EH, J diuide EH into two equal parts in I. Lastly on the Center I with the distance IH, J describe an arch of a circle which must cut the side BC in K. And thus J haue found the lesser of the two meanes, and consequently the greater.